Considering mortality insurance and down a nerding out rabbit hole if anyone wants to jump in

[Catherine_Cullen]

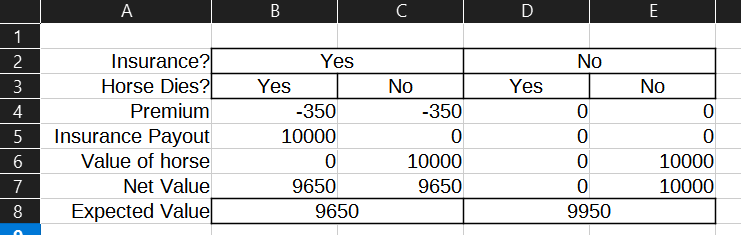
I recently got an online quote from Markel for mortality insurance at 3.5% of the insured value. That seemed like a lot, so I asked Chat GPT to help me figure out the Expected Value for having insurance vs not having insurance. Kind of trying to figure out the cost of taking the risk vs paying the premiums, if you could run the experiment a million times. Does anyone want to take an edible and come along?

So it turns out, and this was not intuitive to me as a non-math person but maybe to others it is obvious, the EV for no insurance and the EV for insurance cross when mortality is .5% of the premium rate. From ChatGPT:

Net Benefit of Insurance vs. No Insurance
Scenario: $10,000 horse value, 3.5% premium rate

Equations:

• EV with insurance:
  EV_ins = (10,000 × p) - 350
• EV without insurance:
  EV_no_ins = -10,000 × p
• Net Benefit:
  Net Benefit = EV_ins - EV_no_ins = 20,000 × p - 350

This shows how much better or worse insurance is compared to going uninsured, based on the mortality probability ( p in decimal form).

Key Insight:

• Net Benefit is zero when p = 0.0175 (1.75%)
• Below 1.75% , insurance has negative net benefit (you’re losing expected value)
• Above 1.75% , insurance has positive net benefit

I’m back. So with a mortality rate of .5x the premium rate, the EV of owning the horse is still negative (lol) but it’s better to have insurance than to not have it. But of course, why would an insurance company sell you that policy? They probably wouldn’t, right? So what should I assume as the expected mortality rate?

The USDA did a study in 2015 that included this table. (side note: RIP federal government and these useful things)

Table 2. Percentage of resident equids that died or
were euthanized in the last 12 months, by age at
death
<6 months: 2.8%
6 months to less than 1 year: 1.2%
1 year to less than 5 years: 0.5%
5 years to less than 20 years: 0.8%
20 years or older: 3.1%

If I plug in a mortality rate of .5% for my hypothetical $10k horse with a $350 premium, the net EV (the difference between the EV of having insurance and the EV of not having it) is -$250. At a mortality rate of .8%, it’s -$190. I thought this was a really interesting way to think about the cost of not risking the loss vs risking it. I know for a lot of people mortality insurance is just the path to medical coverage, so maybe this isn’t useful to them. But just in case someone is interested, here it is!

[Xanthoria]

I have no idea what this means but I support your right to say it.

[2DogsFarm]

Same!

My info is waaaaaay out of date as I haven’t insured a horse since the early 90s.
I only got Mortality coverage because we were traveling to show (commercial shipper) pretty regularly & I considered it Replacement Cost.
At the time I had to provide a Show Record & Training costs to justify the amount I insured my horse for.
For $15K value, IIRC, policy was somewhere around $300 annual premium.
If I’m reading your calculations right, costs haven’t changed much in all those years

As my horse entered his mid-teens, my agent was creative enough (& I wasn’t showing more than 6X a season) to suggest I insure him as a Pleasure Horse.
Likewise suggested insuring DH’s TWH as an Eventer. Neither of us were doing much showing by then.
Because: Percentages & Statistics
How many TBs were insured as Eventers vs TWHs?
How many TWHs were insured as Pleasure Horses vs TBs?

[clanter]

we just insure the horses we have more invested than we can afford to loose, we had one four old who we had to euthanize after a pasture accident, he was insured but to not to his true value just his cost as a weanling.

Currently have two insured a now eight year old mare and a three year old gelding both would be difficult to replace. these are insured at their value.

[Flyaways]

I disagree with ChatGPT’s expected value with insurance. If I understand correctly, this math is saying that if you have insurance and the horse lives, you’re out the $350 premium (so far so good), but if you have insurance and the horse dies, you’re up $9650. I would argue that if the horse dies and you have insurance, you might have $9650 but you also don’t have a horse. Assuming the value of the horse is actually equal to the amount you get from insurance, then if the horse dies you are “made whole” but out the cost of the premium. So I get that the expected values cross when the mortality is equal to the premium rate.

• EV with insurance:
  EV_ins = - 350
• EV without insurance:
  EV_no_ins = -10,000 × p
p = probability of death

(Alternatively, you could say that with insurance you’re up 10k, but without insurance the expected value is 0 because you’re not spending or getting any money regardless of what happens to the horse. my point is just that you have to treat the loss of the horse as -10k in both situations)

To look at it from a purely math perspective, I think it comes down to how much you can get the horse insured for vs. how much it would take to replace that horse. Or if you think your horse has a much larger chance of dying than the average horse.

[Catherine_Cullen]

Oh man, my poor little math brain just exploded.

Here’s the narrative version of what I was trying to get EV to do:

With insurance: you pay the premium, but that doesn’t account for the risk of losing the horse and having the policy pay out.

Without insurance: you pay $0, but that doesn’t account for the risk of losing the horse.

So I was trying to get EV to be a way to put a number on that.

If I write it out un simplified, so it’s ($* probability)+($*probability), then the ChatGPT version was:

EVins: ((10,000-350).005)+(-350.995) = -300
EVnone: (-10,000*.005)+(0*.995)= -50

So for me, that’s giving kind of a useful number to the narrative above.

But I think you’re saying, wait, the insurance scenario isn’t accounting for the loss of the horse. In that .005 scenario, you should be ending up whole (0) in the insurance scenario, not ahead? Like my scenario starts at zero, but it should start at 10,000?

I honestly am not smart enough to figure this out I think. I hear you though that that could help you if you think the value of the horse and the value of the policy are different. I’m hung up on whether the -10,000 in my no insurance ChatGPT scenario is representing the loss of the horse or the cost of not having the policy. I thought it was the loss of the horse, but maybe I’m wrong?

[Flyaways]

@Catherine_Cullen

I’m hung up on whether the -10,000 in my no insurance ChatGPT scenario is representing the loss of the horse or the cost of not having the policy. I thought it was the loss of the horse, but maybe I’m wrong?

You’re right, the -10k in the no insurance scenario represents the loss of the horse. I think there should be -10k to represent the loss of the horse in the insurance scenario as well. So to break it down ($* probability)+($*probability)

EVins: ((10,000-10,000-350) x.005)+(-350x .995) = -350
EVnone: (-10,000x.005)+(0x.995)= -50

(all I did here was take your equation and subtract 10,000 in the insurance, horse dies case). For me it’s easier to write out in terms of a table, and I like positive values:

So with insurance ideally you’re guaranteed to be out the premium in any case. You’re taking a slightly lower expected value than if you hadn’t insured, but you’re avoiding the catastrophic case (cell D7 here). (I guess that fits with my idea of insurance - you as an individual are unlikely to come out ahead in terms of expected value because presumably the insurance companies have better actuarial tables than we do and price their policies so that they stay in business. As individuals we are understandably wary of the catastrophic case and willing to sacrifice a bit of expected value to avoid it. The insurance company (ideally) has enough policyholders that they will make enough money to make up for the claims they have to pay out.)

now if you want to make things even more complicated, my dad would tell us to put the money we would’ve spent on the insurance premium in the stock market…

[Catherine_Cullen]

That makes sense to me!